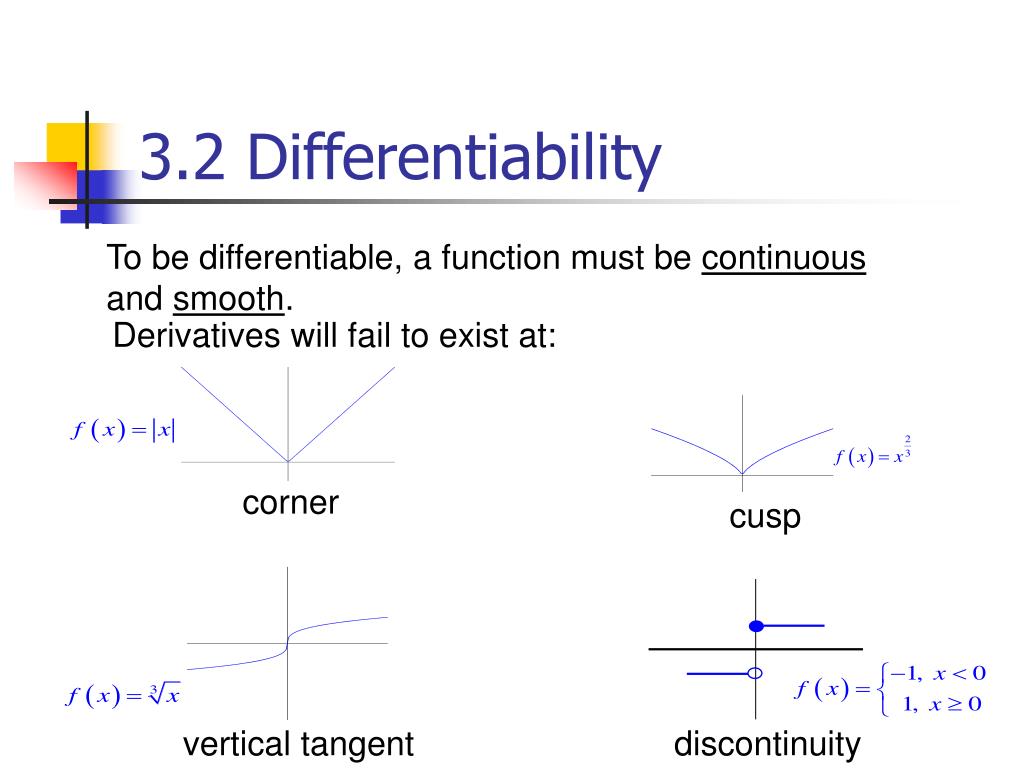
Is A Corner Differentiable . In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. — to determine if a function is differentiable, i first verify its continuity across its entire domain. in summary, a function is not differentiable at places where there is a discontinuity, sharp corner, or an undefined derivative. — a function can be continuous at a point, but not be differentiable there. If a function has a corner or a cusp at a particular point, it is not differentiable at that point. A function f(x) is considered differentiable at a point if. sharp corner or cusp: A function is not differentiable at a point if it has a sharp corner or cusp at that point. That is, up close, the function looks like a.
from www.slideserve.com
— a function can be continuous at a point, but not be differentiable there. In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. in summary, a function is not differentiable at places where there is a discontinuity, sharp corner, or an undefined derivative. sharp corner or cusp: — to determine if a function is differentiable, i first verify its continuity across its entire domain. If a function has a corner or a cusp at a particular point, it is not differentiable at that point. A function is not differentiable at a point if it has a sharp corner or cusp at that point. A function f(x) is considered differentiable at a point if. That is, up close, the function looks like a.
PPT 3.1 Derivative of a Function PowerPoint Presentation, free
Is A Corner Differentiable A function f(x) is considered differentiable at a point if. In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. That is, up close, the function looks like a. — to determine if a function is differentiable, i first verify its continuity across its entire domain. A function is not differentiable at a point if it has a sharp corner or cusp at that point. sharp corner or cusp: — a function can be continuous at a point, but not be differentiable there. in summary, a function is not differentiable at places where there is a discontinuity, sharp corner, or an undefined derivative. A function f(x) is considered differentiable at a point if. If a function has a corner or a cusp at a particular point, it is not differentiable at that point.
From ximera.osu.edu
Geometry of Differentiability Ximera Is A Corner Differentiable That is, up close, the function looks like a. A function is not differentiable at a point if it has a sharp corner or cusp at that point. If a function has a corner or a cusp at a particular point, it is not differentiable at that point. — a function can be continuous at a point, but not. Is A Corner Differentiable.
From www.youtube.com
Where is a Graph Differentiable YouTube Is A Corner Differentiable If a function has a corner or a cusp at a particular point, it is not differentiable at that point. sharp corner or cusp: In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. — a function can be continuous at a point, but not be differentiable there. A function. Is A Corner Differentiable.
From slideplayer.com
Lesson 32 Differentiability ppt download Is A Corner Differentiable In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. sharp corner or cusp: — a function can be continuous at a point, but not be differentiable there. A function f(x) is considered differentiable at a point if. That is, up close, the function looks like a. in summary,. Is A Corner Differentiable.
From www.slideserve.com
PPT BCC.01.9 Continuity and Differentiability of Functions Is A Corner Differentiable A function f(x) is considered differentiable at a point if. A function is not differentiable at a point if it has a sharp corner or cusp at that point. in summary, a function is not differentiable at places where there is a discontinuity, sharp corner, or an undefined derivative. — to determine if a function is differentiable, i. Is A Corner Differentiable.
From www.youtube.com
How To Tell if a Function is Differentiable From a Graph YouTube Is A Corner Differentiable sharp corner or cusp: That is, up close, the function looks like a. If a function has a corner or a cusp at a particular point, it is not differentiable at that point. In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. A function is not differentiable at a point. Is A Corner Differentiable.
From www.slideserve.com
PPT 3.2 Differentiability PowerPoint Presentation ID4636977 Is A Corner Differentiable in summary, a function is not differentiable at places where there is a discontinuity, sharp corner, or an undefined derivative. A function f(x) is considered differentiable at a point if. That is, up close, the function looks like a. — to determine if a function is differentiable, i first verify its continuity across its entire domain. In particular,. Is A Corner Differentiable.
From www.numerade.com
SOLVEDIn Exercises 1116, the function fails to be differentiable at x Is A Corner Differentiable in summary, a function is not differentiable at places where there is a discontinuity, sharp corner, or an undefined derivative. In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. — to determine if a function is differentiable, i first verify its continuity across its entire domain. A function is. Is A Corner Differentiable.
From www.slideserve.com
PPT Differentiability, Local Linearity PowerPoint Presentation, free Is A Corner Differentiable If a function has a corner or a cusp at a particular point, it is not differentiable at that point. A function f(x) is considered differentiable at a point if. A function is not differentiable at a point if it has a sharp corner or cusp at that point. sharp corner or cusp: In particular, a function \(f\) is. Is A Corner Differentiable.
From slideplayer.com
2.1B Derivative Graphs & Differentiability ppt download Is A Corner Differentiable — a function can be continuous at a point, but not be differentiable there. A function f(x) is considered differentiable at a point if. That is, up close, the function looks like a. A function is not differentiable at a point if it has a sharp corner or cusp at that point. — to determine if a function. Is A Corner Differentiable.
From mungfali.com
How To Tell If A Graph Is Differentiable Is A Corner Differentiable If a function has a corner or a cusp at a particular point, it is not differentiable at that point. sharp corner or cusp: — a function can be continuous at a point, but not be differentiable there. In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. —. Is A Corner Differentiable.
From www.toppr.com
Let f R → R be differentiable at c ∈ R and f(c) = 0 . If g(x) = f(x Is A Corner Differentiable A function f(x) is considered differentiable at a point if. A function is not differentiable at a point if it has a sharp corner or cusp at that point. sharp corner or cusp: If a function has a corner or a cusp at a particular point, it is not differentiable at that point. — to determine if a. Is A Corner Differentiable.
From slideplayer.com
The Derivative as a Function ppt download Is A Corner Differentiable If a function has a corner or a cusp at a particular point, it is not differentiable at that point. — a function can be continuous at a point, but not be differentiable there. sharp corner or cusp: In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. —. Is A Corner Differentiable.
From slideplayer.com
Derivatives definition and derivatives of various functions ppt download Is A Corner Differentiable If a function has a corner or a cusp at a particular point, it is not differentiable at that point. — a function can be continuous at a point, but not be differentiable there. A function f(x) is considered differentiable at a point if. A function is not differentiable at a point if it has a sharp corner or. Is A Corner Differentiable.
From www.slideserve.com
PPT The Derivative and the Tangent Line Problem PowerPoint Is A Corner Differentiable — to determine if a function is differentiable, i first verify its continuity across its entire domain. If a function has a corner or a cusp at a particular point, it is not differentiable at that point. A function is not differentiable at a point if it has a sharp corner or cusp at that point. sharp corner. Is A Corner Differentiable.
From www.slideserve.com
PPT 3.2 Differentiability PowerPoint Presentation, free download ID Is A Corner Differentiable sharp corner or cusp: — a function can be continuous at a point, but not be differentiable there. A function f(x) is considered differentiable at a point if. If a function has a corner or a cusp at a particular point, it is not differentiable at that point. — to determine if a function is differentiable, i. Is A Corner Differentiable.
From slideplayer.com
In this chapter, we begin our study of differential calculus. ppt Is A Corner Differentiable — to determine if a function is differentiable, i first verify its continuity across its entire domain. If a function has a corner or a cusp at a particular point, it is not differentiable at that point. In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. A function is not. Is A Corner Differentiable.
From www.slideserve.com
PPT 3.1 Derivative of a Function PowerPoint Presentation, free Is A Corner Differentiable — to determine if a function is differentiable, i first verify its continuity across its entire domain. A function f(x) is considered differentiable at a point if. sharp corner or cusp: In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. A function is not differentiable at a point if. Is A Corner Differentiable.
From www.nagwa.com
Question Video Discussing the Differentiability of a Function at a Is A Corner Differentiable — a function can be continuous at a point, but not be differentiable there. That is, up close, the function looks like a. In particular, a function \(f\) is not differentiable at \(x = a\) if the graph has a sharp. If a function has a corner or a cusp at a particular point, it is not differentiable at. Is A Corner Differentiable.